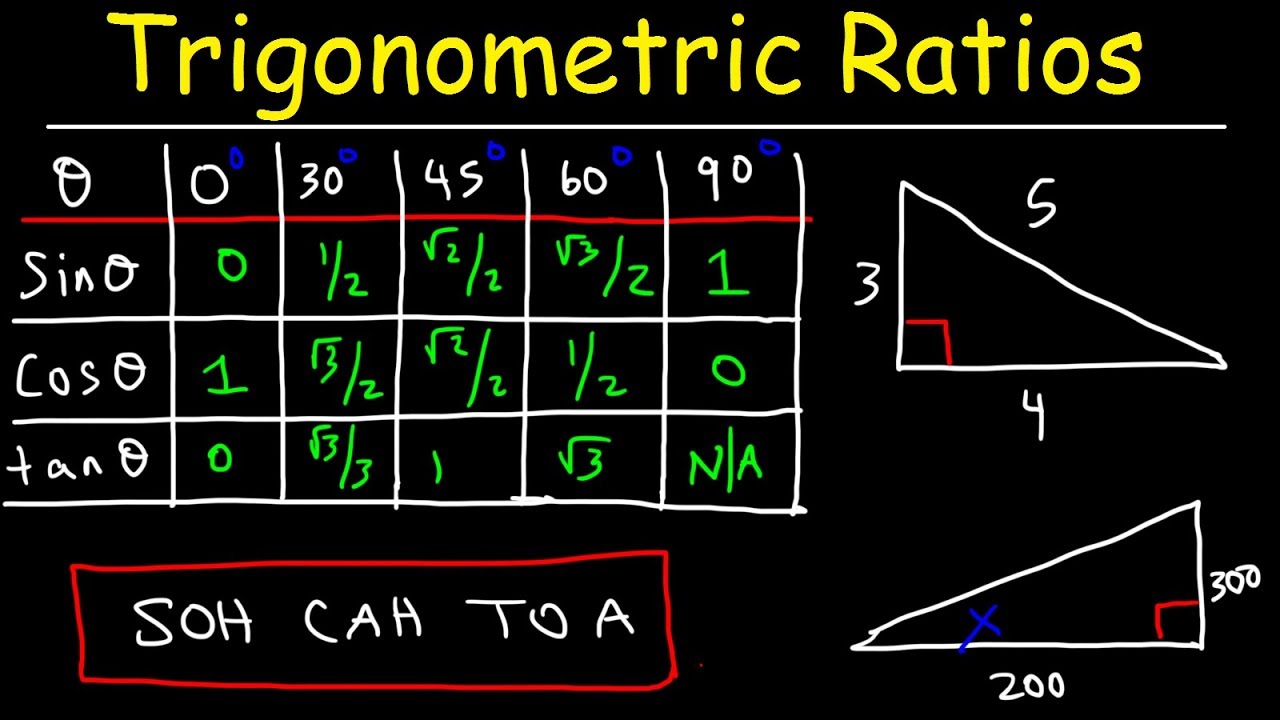
Trigonometry deals with the study of the relationship between the sides and angles of a right-angle triangle. As the name implies, trigonometry refers to the measurements related to a right-angle triangle. The basic trigonometric functions are expressed in terms of the ratios of the dimension of different sides. These are known as trigonometric ratios and are identified by specific names.
Basic Trigonometric Ratios
The six basic trigonometric ratios are obtained by dividing any two of the three sides of the right-angle triangle. The three sides of a right-angled triangle can be denoted as the base, altitude, and hypotenuse. The trigonometric ratios are the set of ratios formed by any two of these sides taken at a time. The ratios are given specific names such as sine, cosine, tangent, secant, cosecant, and cotangent. These are denoted in short forms such as sin, cos, tan, sec, cosec, and cot respectively. Each of these ratios is expressed in terms of the angle over which the function is derived.
Example
Let’s consider a right-angle triangle with base (B), altitude (A), and hypotenuse (H). Let x be the angle opposite to the side (A). Then the trigonometric ratios will be expressed as:
- sin x = Ratio of the altitude to the hypotenuse = A/H
- cos x = Ratio of the base to the hypotenuse = B/H
- tan x = Ratio of the altitude to the base = A/B
- sec x = Ratio of the hypotenuse to the base = H/B
- cosec a = Ratio of the hypotenuse to the altitude = H/A
- cot x = Ratio of base to the altitude = B/A
It is observed from the above that trigonometric ratios denoted by cosec, sec and cot are just inverse of the trigonometric ratios denoted by sin, cos, and tan.
Important Points About Trigonometric Ratios
- The values of the trigonometric ratios don’t depend on the dimension of the sides of the right angle triangle so long the angle remains the same.
- Trigonometric ratios can be used to find out unknown values on any side of a right-angle triangle.
Trigonometric Identities
Trigonometric identities are expressions that involve trigonometric functions and ratios. The trigonometric identities are satisfied by all the values of the variables given in the expression. The different trigonometric identities consist of terms involving the ratios of the side and angles of a right-angle triangle. The trigonometric identity is an equation that holds for any value of the variables to ensure equality of both sides.
Basis of Trigonometric Identities
Any trigonometric identity is based on one or more basic trigonometric ratios which are denoted as sine, cosine, tangent, secant, cosecant, and cotangent. These ratios are obtained by taking ratios of any two sides of a right-angle triangle. The trigonometric ratios are expressed as the function of the angle formed by any two sides of the triangle from the adjacent side, opposite side, and hypotenuse side.
Different Trigonometric Identities
Various trigonometric identities can be used as formulas to solve problems involving trigonometric functions. Some of the trigonometric identities are mentioned:
- Reciprocal trigonometric identities: It represents the three trigonometric ratios (cosec, sec, and cot) as the reciprocal of three other basic trigonometric ratios (sin, cos, and tan) respectively.
- Pythagorean trigonometric identities: These identities are based on the Pythagoras theorem of the right-angle triangle.
- Ratio trigonometric identities: These identities express the tangent and cotangent functions as the ratio of sine and cosine trigonometric functions.
- Complementary and Supplementary angle trigonometric identities: These identities express functions involving complementary angles (the angles having a sum equal to 90 degrees) or supplementary angles (the angles having a sum equal to 180 degrees).
- Double angle trigonometric identities: These identities give the expression of trigonometric functions if the angle value is doubled.
If you are looking to build up your skills in maths from basic to advance, visit Cuemath and book a free session.